At the beginning of the twentieth century, when quantum physics was born, a comprehensive and elegant framework had been developed to explain most everyday occurrences. When one looked very closely, there were a few curious discrepancies between theory and experiment, however. It was through the investigation of these that quantum physics came to light.
It is now understood that the reason these discrepancies were unobserved in everyday life is that quantum physics only becomes apparent when you are dealing with extremely tiny things. Quantum physics is not necessary to describe why the days grow longer in the summer, nor even why grains of sand form a conic shape when piled together. Quantum physics is really only needed when dealing with things even smaller than a speck of dust.
This doesn’t mean that quantum physics is unimportant to our daily lives, however. Recall that physics is used to explain the relationship between matter and energy. All everyday objects are ultimately composed of particles that are much smaller than a speck of dust. Therefore, an understanding of our macroscopic objects (that is, objects of ordinary, everyday size) is inherently reliant on an understanding of their microscopic constituents.

DEFINITION
Macroscopic refers to sizes we are accustomed to dealing with in our ordinary lives (or larger), which can generally be seen by the naked eye.
Microscopic refers to sizes that are much smaller and can only be detected with the help of microscopes or other instruments.
To help put this into perspective, consider the following table. Each number on the left is 10 times smaller than the one above it. The thickness for example, is about 100 times smaller than the height of a human being, and the diameter of a human hair is about 100 times smaller still. As you move to even smaller scales, you enter the domain of quantum physics—waves of light, atoms, and things even smaller.
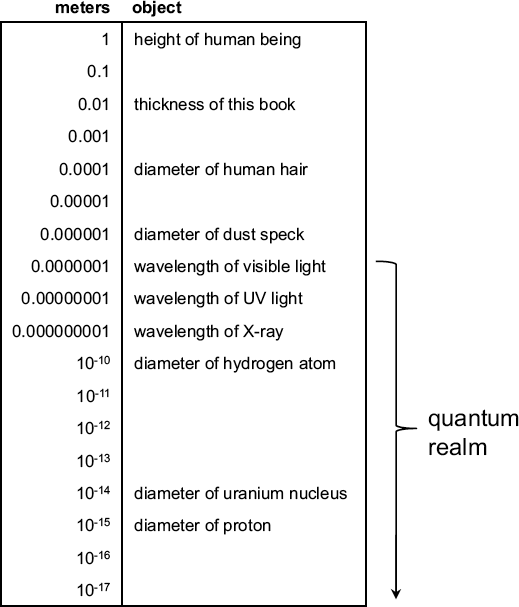
Quantum physics becomes apparent when dealing with exceptionally small things.
We will explain all of these terms in detail, but for now suffice it to say that the effects of quantum physics become truly apparent only on length scales about a million times smaller than you.

ATOM TRAP
In scientific notation, a difference of one unit in the exponent actually corresponds to a factor-of-ten difference in overall magnitude. For example, 1 × 104 is one hundred times larger than 1 × 102. It is this very compression that makes scientific notation so useful when considering values over a broad range.
Here, we have used two types of numbers in the left-hand column. The first 10 entries are written in decimal form, where one additional zero is inserted for every factor-of-ten reduction in size. The next eight entries are written using scientific notation. In general, a number in scientific notation (say, M × 10N) can be written in decimal form by using the correct number of zeroes and the value M. When N is a positive number, you insert N zeros to the left of the decimal, and when N is negative you insert (N – 1) zeroes to the right. For example, 5 × 102 = 500 while 5 × 10-3 = 0.005. Also, if there is no number M specified, this means that it is simply equal to one. In other words, the number 10-10 is equivalent to 1 × 10-10.
What’s New?
Quantum physics is just a little over 100 years old. Its emergence at the beginning of the twentieth century was driven primarily by physicists’ ability to finally observe, manipulate, and measure objects down at the quantum scale. What they found was that the microscopic world was not just a scaled-down version of the macroscopic world. Instead, it is fundamentally different, and governed by new physical laws that are actually quite strange. When you boil it all down, there are about four new ideas that emerged with quantum physics. We’ll cover all of these in due time, of course, but we summarize them briefly here.
Leave a Reply