We will introduce new concepts by describing how they were originally discovered. Unfortunately, in the quantum world, many of these concepts are not the most straightforward and easy to understand. To get off to a good start, then, let’s first get a feeling for the process of physics by considering a familiar, everyday occurrence.
Imagine that you are seated comfortably in King Arthur’s court, near enough to the Round Table that you can hear what’s going on. It is early spring, and King Arthur and Sir Lancelot are discussing their observation that the days are getting longer. Arthur has been studying this carefully, and has noticed that the amount of daylight increases by about the same amount each day. As a result, he concludes that the days will continue to lengthen by the same amount for all days going forward. He even comes up with a mathematical model to describe this: H = At. Here “H” is the number of hours of daylight, “t” represents time in days, and “A” is just a constant number that quantifies how much longer each day is compared to the last. (Most physics formulas require physical constants like “A” to make the mathematical relationships quantitatively agree with observation.)
“Nonsense,” says Lancelot. Thinking back to the previous autumn, when the days were actually getting shorter, he reasons instead that there must be a cyclical effect at play. He proposes an alternative formula to describe the number of hours of daylight based on a simple trigonometric sine function (a sinusoid).
Using top-of-the-line hourglasses, Arthur and Lancelot carefully measure the number of hours of daylight every Saturday for two months, and then plot this on a graph. From this graph, Arthur and Lancelot make a few tweaks to their formulas to bring them into agreement with observation. (This is when Arthur selects the value “A” needed so that his formula will match the data.) With only two months’ worth of observations, both formulas agree well enough with the data that both knights are feeling rather confident.
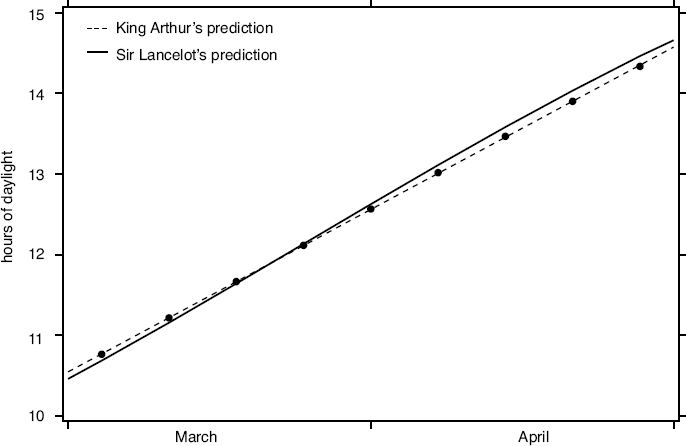
After two months, both King Arthur’s and Sir Lancelot’s models demonstrate good agreement with the observed values (given by black circles).
Arthur is called off on an adventure, during which he is away for 10 months. When he finally rides over the drawbridge back into Camelot, Lancelot can’t help but gloat. A look at how their formulas compare to observation explains why. When more data are included, Lancelot’s model turned out to be right on, while Arthur’s was way off the mark. As a result, Lancelot’s formula is accepted, while Arthur’s formula is discarded.
With new confidence in Sir Lancelot’s formula, Merlin (our theorist) retires to his chamber to come up with an explanation for why it works in the first place. After some careful thinking and mathematical modeling of his own, he concludes that not only does Earth revolve about its axis, but that the axis of rotation must be tilted at an angle relative to the plane of its orbit about the sun. As Earth orbits The Sun, its northern hemisphere is tilted toward the sun during the summer and away during the winter. Therefore, the days grow longer in the spring and shorter in the fall.
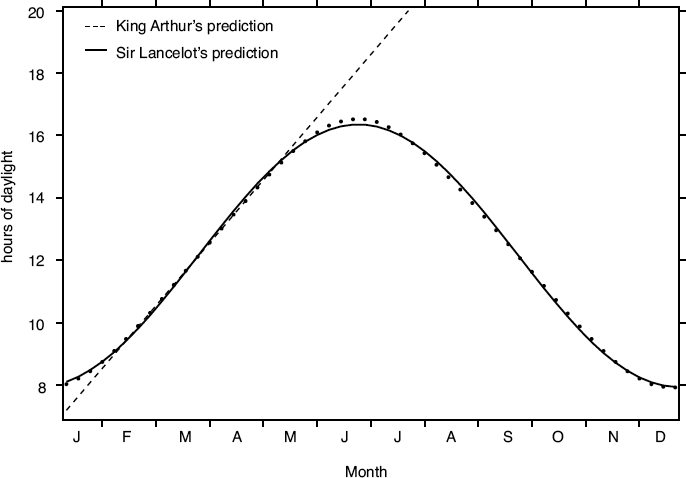
After a year, Sir Lancelot’s theory is validated while King Arthur’s is rejected.
A careful look at the data, however, reveals that even Lancelot’s prediction isn’t perfect. For example, in June and July it predicts the days should be a smidge shorter than they actually are. Lancelot’s formula and Merlin’s interpretation require a little fine-tuning to account for subtle effects and bring it into better agreement with observation. Nevertheless, the Lancelot-Merlin theory is a very good approximation of the length of the day throughout the year. It can even be used to predict day length for many years to come. Based on its success, this theory is elevated to the status of physical law.
Though this example is fictitious, the process through which king arthur, sir Lancelot, and Merlin tried to describe nature is typical of the way physics is actually done. A certain phenomenon is observed, and then theorists derive formulas and interpretations to explain it. Experimentalists make careful measurements, and based on these, successful theories are validated and unsuccessful theories are discarded. Theories that stand the test of time are considered to be physical laws.
Meanwhile, minor discrepancies between successful theories and experiment are carefully studied to see what refinements are needed to bring theory into even better agreement with experiment. Typically, a little fine-tuning is all that’s needed, and it usually involves accounting for subtle effects that are consistent with the theory in question. But in some cases, a slight disagreement between theory and experiment turns out to reveal fundamental flaws in the theory and ultimately lead to the emergence of revolutionary new ideas. This, as we’ll see, was the case for quantum physics.
Leave a Reply