Mass Moment of Inertia of Sphere

Consider an elemental plate of thickness dy at a distance y from the diametral axis as shown in Figure 11.24. Radius of this elemental circular plate x is given by,

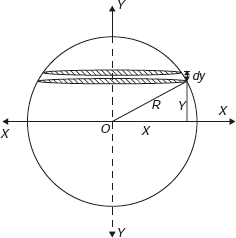
Figure 11.24 Mass Moment of Inertia of Sphere

x² = R² − y²

 

Mass of the elemental plate, dm = ρπx² dy = ρπ (R² − y²)dy

Moment of inertia of the plate element about axis

But, mass of sphere,

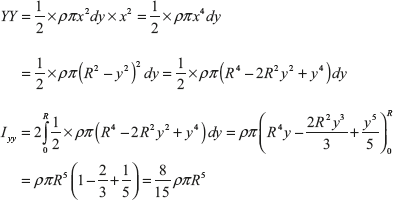
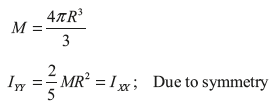
Mass moment of inertia of hemisphere about the axis ZZ,

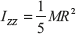
Moment of inertia of the plane element about the axis