Mass Moment of Inertia of a Hollow Cylinder

Let in Figure 11.23, R_o = Outer radius of cylinder; R_i = Inner radius of the cylinder, L = Length of cylinder; ρ = Mass density of the cylinder; and M = Mass of the cylinder.

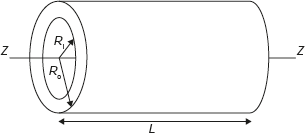
Figure 11.23 Mass Moment of Inertia of a Hollow Cylinder

Consider a small elemental ring of width dr at a distance of radius r from the centre of cylinder then the mass of the element, dm = ρ·2πr·dr·L

Now mass moment of inertia of the element about the axis ZZ

I_ZZelement = (ρ × 2πrdr × L)× r²

Moment of inertia of the cylinder about the axis ZZ