Heat Transfer Through Sphere

Consider a hollow sphere of internal radius r₁ and external radius r₂ as shown in Figure 7.8. Let the inside and outside surface temperature be t₁ and t₂; and let the thermal conductivity be k. Consider a small element of thickness dr at any radius r. It can be shown that the surface area of this spherical element is given by 4πr². The heat transfer rate

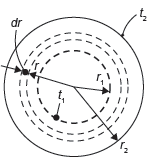
Figure 7.8 Heat Transfer through Sphere

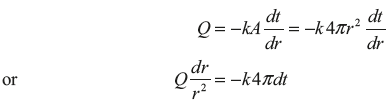
On integrating, we get

Applying electrical analogy, we get

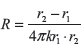
If the concept of mean area, A_m and mean radius, r_m are applied

where

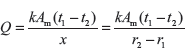
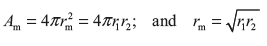
r_m is geometric mean.

Example 7.6: A small hemispherical oven is built of an inner layer of insulating firebrick 120 mm thick and an outer covering 80% magnesia 40 mm thick. The inner surface of the oven is 820°C and the heat transfer coefficient for the outside surface is 10 W/m²K; the room temperature is 22°C. Calculate the heat loss through the hemisphere, if the inside radius is 0.8 m. Take the thermal conductivities of firebrick and 80% magnesia as 0.31 and 0.05 W/m K.

Solution:

Given: