When velocity of the belt is more than 10 m/s, the centrifugal force becomes predominant. Analyse the various components of forces as shown in Figure 15.6.
Let ρ is the density of belt materials
Tc is centrifugal tension on the belt element in tight and slack side
r is radius of the pulley
t is thickness of the belt
b is width of the belt
σ is maximum allowable stress in the belt
m is mass per unit length of the belt
FC is the centrifugal force on the element
V is the velocity of the belt
Δθ is the angle of lap
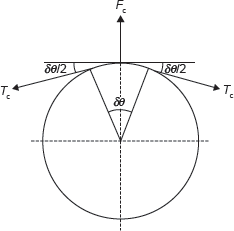
Figure 15.6 Centrifugal Force in Belt Drive
Now,
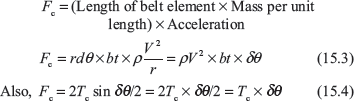
From Eqs. (15.3) and (15.4), we get
Tc = ρ (bt) V2
Total tension on tight side,
T = T1 + Tc,
where T is maximum allowable tension equal to σ × b × t
Total tension on slack side = T2 + Tc
Example 15.4: A leather belt of density 1,000 kg/m3, thickness 10 mm is used to transmit a power of 8 kW from a pulley 1.5 m in diameter running at 300 rpm. Determine the width of the belt taking centrifugal tension into account. If angle of lap is 165° and coefficient of friction between belt and pulley is 0.25. Assuming allowable stress for leather belt is 1.5 MPa.
Solution:
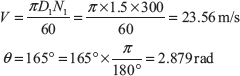
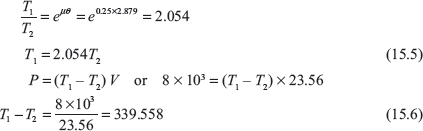
From Eqs (15.5) and (15.6), we get
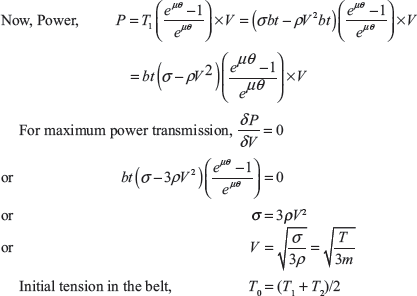
Example 15.5: An open-belt drive transmits a power of 3.0 kW. The linear velocity of the belt is 3 m/s. Angle of lap on smaller pulley is 160°. The coefficient of friction between belt and pulley is 0.25. Determine the effect on power transmission in the following cases:
- Initial tension in belt is increased by 10%.
- Angle of lap is increased by 10% using idler pulley for the same speed and tension in tight side.
- Coefficient of friction is increased by 10% for same initial tension.
Solution:
T1 is the same as before whereas θ is increased by 10%
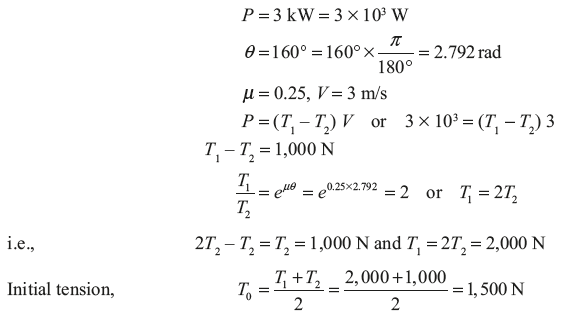
Leave a Reply