Exposure Assessment

Measures of ongoing exposure to health risks call for determination of concentration. In the Section 5.1.3.1, Example 5.3 shows how to determine bioconcentration, and Example 5.4 explains the use of slope factors, often used in quantifying risks of cancer.

Partition Coefficient and Bioconcentration Factor

While extensive research continues to refine existing methods and to develop new markers of exposure and effect, experience has identified several biomarkers of value to hazardous waste management decisions. Exposure to chemicals that bioaccumulate in tissue (see, e.g., Table 5.2) is easily accomplished by analyzing tissue residue. In the absence of tissue residues, empirical constants often derived and used by ecotoxicologists to predict bioaccumulation include the octanol–water partition coefficient (K_ow), which approximates a chemical’s behavior between the lipid water phase and the bioconcentration factor (BCF), which describes the relationship between the chemical concentration in an organism and the concentration in the exposure medium (typically water). The BCF is empirically expressed as

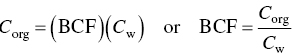
Example 5.3 illustrates the use of this relationship.

EXAMPLE 5.3 PREDICTING BIOCONCENTRATION

Routine monitoring of sediments and surface water downstream from a hazardous waste site indicates that reportable concentrations of the pesticide DDT average, respectively, 33 mg/kg and 0.25 μg/l. Considering that the US Food and Drug Administration has identified levels of 5 ppm or higher of DDT as requiring action, is there a reason to be concerned about potential accumulation of DDT in fish tissue near this site?

SOLUTION

Since the routine monitoring does not provide information regarding DDT residues in fish tissue, we refer to Table 5.2 and BCF. Transforming the log BCF of 4.78, we estimate a BCF of 60.256 (mg/kg DDT in tissue per mg/l DDT in water or l/kg).

Table 5.2 Selected physicochemical parameters and bioconcentration factors for typical compounds which bioaccumulate.

Compound	Log Kow	Log BCF (fish)	Log Koc	S (mg/l)
Chlordane	5.15	4.05	4.32	0.0056
DDT	5.98	4.78	4.38	0.0017
Dieldrin	5.48	3.76	4.55	0.022
Lindane	4.82	2.51	2.96	0.150
Chlorpyrifos	4.99	2.65	4.13	0.3
Cyhexatin	5.38	2.79	<3.64	<1.0
2,4D	1.57	1.30	1.30	900
2,4,5T	0.60	1.63	1.72	2.38
TCDD	6.15	4.73	5.67	0.0002

BCF, bioconcentration factor (l/kg); K_oc, organic carbon partition coefficient; K_ow, octanol–water partition coefficient (unitless); S, water solubility.

Substituting our monitoring data and the empirical BCF for DDT in the following equation yields

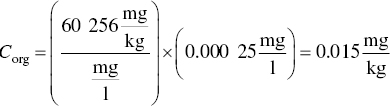
We estimate a DDT tissue residue of 0.015 mg/kg. Based on this estimate, it is probable that DDT residues in tissue are an issue that should be evaluated.

The calculation of carcinogenic risk involves the use of a carcinogen potency factor (CPF). A CPF is basically the slope or steepness of the dose–response curve at very low exposures, and is now referred to as slope factor. The dimensions of a slope factor are expressed as the reciprocal of the daily dose (mg/kg/day)⁻¹. Having derived a slope factor, the calculation of carcinogenic risk is straightforward. Quantification of carcinogenic risk of an exposure simply requires converting the dose to the appropriate terms (mg/kg/day) and multiplying it by the slope factor as demonstrated in Example 5.4.

EXAMPLE 5.4 USE OF SLOPE FACTORS

A petroleum refinery is performing a quantitative risk assessment on the atmospheric release of volatile organic compounds (VOCs) from the facility, some of which are toxic. Assess the risk of benzene released to the air. What is the maximal number of excess lifetime cancer cases expected for a population of 100 000 adults with a daily inhalation dose of 0.14 mg of benzene per adult body weight per day?

SOLUTION

1. Inhalation carcinogenic risk:
2. The slope factor for benzene is 0.029 (mg/kg BW‐day)⁻¹ taken.
3. Assuming an adult weight of 70 kg, the lifetime cancer risk is calculated as

Exposure Point Concentrations

The risk analyst must next estimate the concentration of contaminants at the exposure points, which define the locations of the receptors, that is the human or animal population whose exposure to a pollutant is of concern. Receptors are identified for each exposure scenario simply by overlaying the demographic information with the exposure pathway. An exposure point may be as close as the sources of waste at the site itself (e.g. the trespasser scenario) or at a considerable distance, particularly for pathways involving the food chain. It may take any pathway: air, ground and surface water, soils, sediments, and food (e.g. plants and fish). For present exposures, actual monitoring data at the exposure point should be used wherever possible. For example, contaminant concentrations should be obtained for drinking water wells in the vicinity of a site. For on‐site exposure points, representative concentrations in soil or groundwater may be calculated as the arithmetic or geometric mean (depending on the statistical distribution of the site analytical concentration data).

Future conditions can differ starkly. A plume of gases may not have yet migrated to a potential exposure point. Remediation will, of course, drastically reduce migration. In a comprehensive risk assessment, the exposure concentrations for each for remedial alternative would be estimated including the present situation (baseline conditions of no remedial action). Determining the concentration of a contaminant at the exposure point for future conditions often requires the use of fate and transport modeling methods and standard references. The major effort with these models is calibration; once calibrated, successive runs can be made relatively easily to estimate concentrations for a range of conditions and assumptions.

For groundwater contaminants, hydrogeologic models can be used to estimate the future concentration at a downstream well. For VOCs released to the atmosphere, a Gaussian diffusion model can be employed to estimate future downwind concentrations. In general, the level of effort employed in data collection and modeling will depend on the estimated severity of the risk. Nominal risks do not warrant the same level of analysis as the clearly significant risks.

All mathematical models require the making of assumptions. This is true, as well, of the mechanistic models on which many analyses are based.