Working of Impulse Turbine

In the impulse turbine, all the pressure drops occur in nozzle and there is no pressure drop of steam passing through blades. Let us consider steam enters the nozzle with pressure of P₀ and velocity of V₀, after expansion of steam in nozzle pressure drops to P₁ and velocity increases to V₁. High velocity jet of steam impinges on the blades with velocity V₁ gets deflected by an angle and comes out with smaller velocity V₂ producing an impulse on the blades. The pressure P₁ remains constant passing through the blades.

Now, momentum at the inlet of the blade – Momentum at the exit of the blade = Impulse on the blade absorbed in producing shaft work.

After expansion of steam in nozzle, it strikes the blades with absolute velocity V₁ which rotates the blade with mean peripheral velocity u, steam leaves the blade with relative velocity V_r2 and absolute velocity V₂ as shown in Figure 5.9.

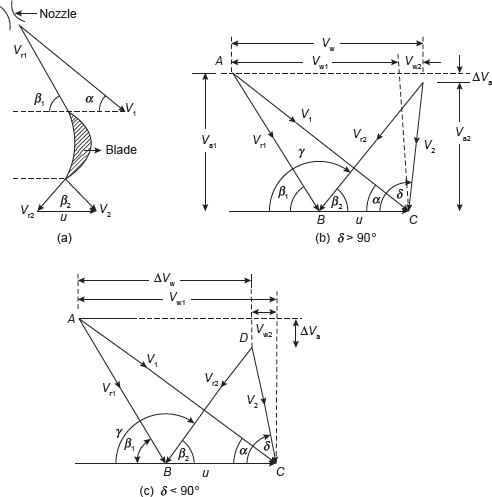
Figure 5.9 Velocity Diagram for Impulse Turbine

Here,

In Figure 5.9b, in ΔABC

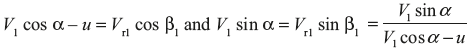
In ΔCBD

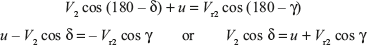
Similarly, in Figure 5.9c

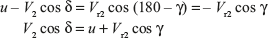
Blade Friction Factor: It is ratio of relative velocity of steam at exit to the relative velocity at inlet of the blade. It is denoted by K_b

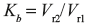
Energy loss due to friction in blade

In terms of K_b, ΔV_ω = (V₁ cos α – u) (1 + K_b); if blade are symmetrical, i.e., β₁ = β₂

Tangential Thrust

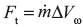
Axial Thrust

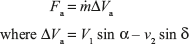
Blade Work or Diagram Work

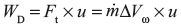
Input energy to the blade = 

Blade or Diagram Efficiency

Maximum Blade Efficiency and Optimum Velocity Ratio: Substituting ΔV_ω in equation of blade efficiency

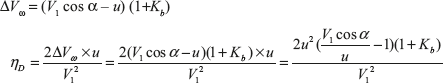
Velocity ratio is the ratio of mean velocity of blade to the jet velocity

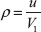
as

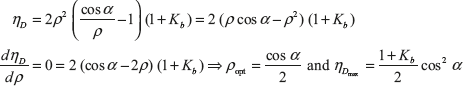
Putting the value of ρ as  and if loss of energy due to friction is zero, i.e., K_b = 1

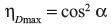
Thus, lower is the nozzle angle, higher is the blade efficiency. But, too low nozzle angle causes energy loss at the blade inlet. Therefore, the nozzle angle is to be maintained within certain range which varies from 16° to 22°.

Example 5.1: Steam enters an impulse turbine at 1,000 m/s and at a nozzle angle of 18°. The mean peripheral velocity of blades is 600 m/s and blades are symmetrical. If the steam is to enter the blades without shock, what will be the blade angles?

1. If friction effect is negligible on the blades, calculate the tangential thrust on the blades and diagram power for unit mass flow rate of steam.
2. If blade friction (Kb) is 0.8, estimate the axial thrust, diagram power and diagram efficiency.

Solution:

Velocity triangle is shown in Figure 5.10.

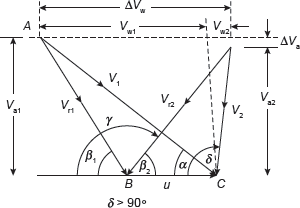
Figure 5.10 Velocity Triangle

1. From Eq. (5.1), 
2.